In most foreign codes, the methods for PCRD can be classified into 3 major types: Conceptual method, Tie Force (TF) method and Alternate Path (AP) method (Liang et al., 2007a).

Conceptual method is an indirect design approach. It requires the enhancement on the integrity, ductility and redundancy of structures by rationally arranging structural members and strengthening weak members and joints. But this method greatly depends on engineers¡¯ experience.

Tie force (TF) method is also an indirect design approach that enhances the continuity of the structural elements by requiring tie strength to guarantee the integrity of structures and reserved load path. It¡¯s convenient to implement because it does not need to calculate the response of the whole structure. However, as too many assumptions have been set to build up this method, the empirical factors are needed to be carefully checked to guarantee the safety of TF method.

Alternate path (AP) method is a direct design method to guarantee that the structure is capable of bridging over a removed structural element so as to prevent the damage from exceeding beyond the limits. If there is a structural element that can not be bridged over, this element must be designed as a key element, which should have enough strength to resist possible extreme loads.

2.2 Design Strategies

The reliability, complexity and workload of the three methods mentioned above obviously increases in order. And therefore, the foreign codes categorize building structures according to their protection level, as seen in Table 1, and suggest proper methods for each protection level.

3.2.1 Design Procedure

The general design procedure for LSAP method is as follows:

1. Remove target vertical structural elements one-by-one and conduct a LS analysis for each removing to get the static design internal force S_static;

2. Approximately estimate the dynamic design internal force S_dynamic with the dynamic amplification factor A:

S_dynamic=A S_static          (1)

3. The elasto-plastic strength requirement of residual structural elements R can be calculate by

R¡Ýb¡¤ S_dynamic         (2)

in which b is the strength reduction factor that represents the energy dissipation capacity in plastic deformation of real structures.

So from above procedure, it can be found that the two major simplifications of LSAP method, which are the static approach and the linear approach, are represented with dynamic amplification A factor and strengthen reduction factor b, respectively. These two factors need to be determined by comparing the difference between nonlinear dynamic results and linear elastic results.

3.2.2 Design Factors

A typical Chinese 3-storeys concrete frame is analyzed with linear static analysis, linear dynamic analysis and nonlinear dynamic analysis to determine suitable values of design factors mentioned above. It is found that A=2.0 and strength reduction factor b=0.67 is rational and safe (Figure 5 and Figure 6).

The proposed procedure and factors for LSAP design is checked with the 8-storey RC frame in former chapter. The structure avoids progressive collapse, which proofs the effect of proposed LSAP method. And material cost is listed in Table 4 which shows that the proposed LSAP method is safe and very close to the precise value from NDAP design.

4 tie force method

Comparing to AP method, Tie Force (TF) method does not calculate the response of the whole structure. On the contrary, it treats the whole structures as the combination of many substructures (Figure 7) and assumes that if every substructure has proper strength and enough deformation capacity, the whole structure can avoid the collapse. Besides above, the TF method directly analyzes the ultimate state of each substructure thus the substructure can be treated as a static determinate problem (Figure 8). So TF method is much easier than AP method but also has lower precision.

4.1 Basic Assumptions

1. The tied elements bridge over a vertical structural element by two mechanisms, beam mechanism with bending strength (Figure 9) and catenary mechanism (Figure 10) with tensile strength.

2. For the beam mechanism, only bending capacity at the fixed end of the beams are considered (Figure 9).

3. The catenary mechanism of beams is considered only if the beams are continuous and pass through the joint.

4.2 Design Procedure

1. Build up the substructure model as shown in Figure 7 according to their locations in the building.

2. Transform distributed loads and concentrated loads on each beam to an equivalent concentrated load N¡¯ at the joint, which results in an equal bending moment to the fixed end of each beam. Sum N¡¯ of every beam in the substructure to obtain the total equivalent concentrated load N.  

3. N will be borne by the resistance of beam mechanism (Figure 9) and catenary mechanism (Figure 10).

         (3)

where: i is the serial number of beam, n is the total number of beams in the substructure,  and  are the resistances of i^th beam with beam mechanism and catenary mechanism, respectively.

4. The total resistance of beam mechanism can be calculated:

          (4)

where: L_i is the length of i^th beam,  is the negative bending capacity at the fixed end of i^th beam.

5. If N can be borne by beam mechanism, the tie force does not need to be considered. Otherwise, the total vertical resistance from tie force is given by:

        (5)

And if there is more than one direct in which there are beams continuously pass through the joint, the tie force in each direction can be obtained according to the bending stiffness of beams in each direction:

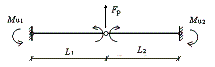
         (6)

where: j is the direction number, I_j is the rotational inertia of beams in j^th direction,  is the tie force of beams in j^th direction.

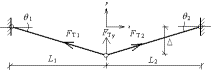
Finally, in j^th direction, the tie forc of beam can be obtained from the balance of horizontal tie force at the joint:

         (7)

         (8)

where:  and L_j1 are the tie force and the length of the first beam in j^th direction, and L_j2 are the tie force and the length of the second beam in j^th direction.

4.3 Application and Verification

The former 8-storey frame is redesigned by TF method proposed by this paper. There are three kinds of substructures in the structure, as seen in Figure. 7. For the interior substructures, four beams provide tie forces in both two directions because of their continuities (Figure. 7a). On the contrast, only two beams provide tie forces for the side substructures (Figure. 7b). And both interior and side substructures can provide beam mechanism and catenary mechanism to prevent collapse. However, for corner substructure (Figure. 7c), only beam mechanism can provide the collapse resistance capacity.

The 8-storeys frame after TF design satisfies the requirement of progressive collapse resistance, and consumption of longitudinal steel bars is also listed in Table 2. It can be concluded that TF method in this paper is effective.

5 conclusions

AP method and TF method are feasible for engineering design. NDAP method is the most accurate methods but with high difficulty and complexity, so it is suitable for very important and complicated structures. On the contrary, LSAP method and TF method are easier to implement and their accuracy are guaranteed by carefully choosing the factors and computational models. Based on a series of rigorous researches, this paper suggests empirical factors, computational models and design procedures of LSAP method and TF method on progressive collapse resistance design for Chinese engineers.

ReferenceS

DoD. (2005). UFC 4-023-03: Design of Structures to Resist Progressive Collapse.

Ellingwood, B. R. (2006). Mitigating Risk from Abnormal Loads and Progressive Collapse. Journal of Performance of Constructed Facilities, 20:4,315-323.

EuroCode 1. (2005). Actions on structures.Part 1.7: General Actions - Accidental actions,  European Committee for Standardization.

GB50010-2002. (2002). Code for Design of Concrete Structures, Beijing: China Building Industry Press, China.

Liang, Y., Lu, X.Z., Miao, Z.W., Ye, L.P. (2007a). Progressive Collapse of Structures: Introduction and Comparison of Standards. Proc. 6th National Conference on Safety Protection for Engineering Structures, Luoyang, China, 195-200. (in Chinese)

Liang, Y., Lu, X.Z., Li, Y., Ye, L.P., Jiang, J.J. (2007b). Design Method to Resist Progressive Collapse for a Three-story RC Frame. Journal of PLA University of Science and Technology, 8:6, 659-664. (in Chinese)

Lu, X.Z., Zhang, Y.S., Jiang, J.J. (2007a). Simulation for the Collapse of Reinforced Concrete Frame under Blast Load with Fiber Model, Blasting, 24:2, 1-6. (in Chinese)

Lu, X.Z., Li, Y., Miao, Z.W., Chen, X.P., Liang, Y. (2007b). High Performance Simulation for Building Structures under Disaster. Proc. 1st Symp on High Performance Computing (HPC) Technology in Engineering Design. Shanghai, China, 93-101. (in Chinese)

Wang, X.L., Lu, X.Z., Ye, L.P. (2007). Numerical Simulation for the Hysteresis Behavior of RC Columns under Cyclic Loads. Engineering Mechanics, 4:12, 76-81.

Yi, W.J., He, Q.F., Xiao, Y. (2007). Collapse Performance of RC Frame Structure. Journal of Building Structures, 28:5, 104-109. (in Chinese)