1 Introduction
In recent years, there has been a rapid development in high-speed rail construction worldwide. Such a development of railway infrastructure is often accompanied by the severe derailment accidents. Over-speed, impractical railway safety measures, poor weather conditions, and mechanical problems are common causes of train derailment accidents in many countries [1]. These accidents may cause significant casualties and property losses (Figure 1). Derailed high-speed trains, in particular, can collide with surrounding structures, leading to severe secondary disasters. The Amagasaki rail crash was a typical accident. In 2005, having failed to slow down on a curved track properly, five carriages of a high-speed train suddenly derailed on the JR West Fukuchiyama Line in Amagasaki, just in front of the Amagasaki Station on the way to D¨shisha-mae. The train rushed into a residential building after colliding with a car. This accident killed 107 people and injured 562 others, according to Wikipedia [2].
|
|
|
|
(a) A German train derailed causing bridge collapse in 1998 [3]. |
(b) A Japanese train derailed and collided with high-rise building in 2005 [4]. |
|
|
|
|
(c) A Norway train derailed and collided with a building in 2010 [5] |
(d) An Argentine train derailed and collided with station in 2012 [6] |
|
|
|
|
(e) A France train derailed and crashed into platform in 2013 [7] |
(f) A USA New Jersey commuter train derailed and crashed into platform in 2016 [8] |
|
Figure 1 Train derailment accidents occurred in various countries |
|
In addition to the Amagasaki rail crash, there have been many collision accidents of derailed trains with buildings or bridges, as listed in Table 1. It has become evident that the secondary disasters caused by the collision of a derailed train with buildings or bridges near the tracks are more severe than the train derailment itself. The corresponding collision risk, collision pattern and collision load are closely related to the running attitude of the train after derailments.
Problems associated with train derailment have received increased attention since Nadal [9] firstly proposed the criterion for derailments. Brabie [1] systematically reviewed the relevant studies on the derailment of high-speed trains. In recent years, real accident analyses and early detections of train derailment have been intensively studied [10-12]. However, research to date was primarily focused on how to prevent and monitor train derailments. In contrast, studies of the running attitude of trains after derailment are very limited.
Table 1 Derailed trains collide with buildings leading to secondary disasters
|
Date |
Location |
Cause of derailment |
Casualties |
Building or bridge damage |
|
June 1998 |
Eschede, near Hanover, Germany |
The train tires are broken. |
101 people were killed and 88 wounded. |
Break the bridge pier, causing the main bridge collapse. |
|
April 2005 |
Fukuchiyama Line in Amagasaki, Japan |
The train is over-speed in the corners. |
107 people were killed and 562 wounded. |
The collision caused serious damage to the residential building. |
|
March 2010 |
Oslo, Norway |
No traction locomotive, the train slides out of the track |
3 people were killed and 3 wounded. |
A building collapsed after the derailed trains collide with the building. |
|
February 2012 |
Buenos Aires, Argentina |
Turnout operation error and brake failure |
50 people were killed and 703 wounded. |
The derailed train collided with the platform. |
|
July 2013 |
Paris, France |
Railway parts are broken. |
6 people were killed and 22 wounded. |
The derailed train crashed into the platform and damaged the platform. |
|
September 2016 |
New Jersey, USA |
Train speeding |
1 people were killed and 114 wounded |
Collapsed portions of the station roof after the crash. |
Therefore, an in-depth study of the running attitude of a train after derailment is essential to improve the design, operation, and maintenance of railway systems. The work presents a numerical simulation of the running attitude of a train after derailment based on nonlinear finite element analysis. Using the simulation results, the running attitude of the train after derailment was analyzed with the goal of preventing the secondary disasters caused by derailed trains.
2 Finite element model
2.1 Track model
A key issue when simulating the running attitude of a train after derailment is how to simulate the contact between the derailed train wheels, carriages, tracks and the ground (including the concrete sleeper). Hence, this study used the commercial finite element software package MSC.MARC, which is widely used in contact computation to establish a finite element model [13]. Xu et al. [13-14] have analyzed the collision between over-height truck and bridge superstructure using MSC.Marc. The method of establishing the train model in this work is similar to that Xu et al.¡¯s work [13-14]. In constructing the models, solid elements are used for all main contact bodies mentioned above to ensure the accuracy and reliability of contact simulation. The contact module of MSC.Marc is utilized to definite the contact between different components. Specifically, the contact between the train body, the wheelset, the track and the ground were defined separately. The contact table of MSC.Marc defines the coefficients of friction between the train body, the wheelset, the track and the ground. For the normal contact condition, the contact distance tolerance is set to be zero to avoid any penetrability. For the tangential contact condition (i.e., the friction condition), MSC.Marc provides an arctangent model instead of the Coulomb friction model to improve the convergence efficiency [15]. The expression of which is shown in Equation (1).
|
|
(1) |
where t is the distance of tangential relative sliding. The value of RVCNST can be seen as the value of the relative velocity below which sticking occurs. The value of RVCNST is important in determining how closely the mathematical model represents the step function.
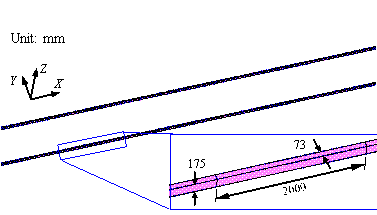
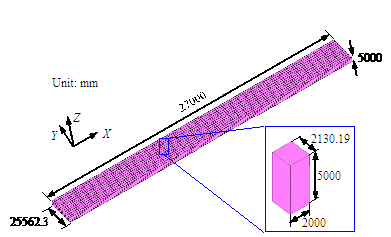
Specifically, the tracks are simulated as a steel material based on an ideal elastic-plastic material according to the von Mises criterion. The concrete sleeper and the ground under the tracks are simulated as concrete material, which is also based on an elastic-plastic material model [16]. The finite element models of the tracks, the concrete sleeper and the ground are shown in Figures 2 and 3, respectively. The shapes of tracks are determined according to their actual sizes. Ballastless tracks are used in this simulation. The tracks and ground are connected through sharing nodes in the finite element model. Han & Koo [17] and Bae et al. [18] showed that the simplification of the tracks has little effect on calculation results.
|
|
|
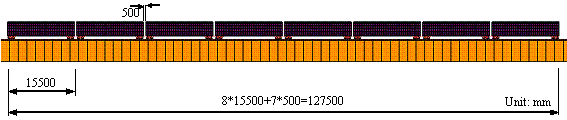
Figure 2 Finite element model of the tracks (unit: mm) |
|
|
|
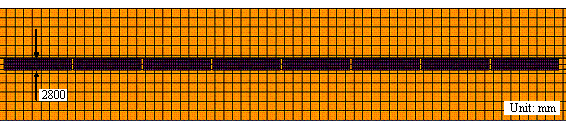
Figure 3 Finite element model of the ground (unit: mm) |
2.2 Finite element model of the train
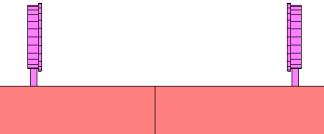
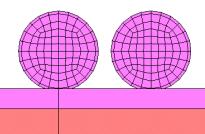
The finite element model of the train has a significant influence on the computational results. To obtain representative results, the parameters of the train model are determined according to a typical Chinese-manufactured train that has eight carriages. The running speed of the train during the derailment accident is set to 80 km/h, which is the highest speed limit in China for a train arriving at the station at the turnout. Hence, the simulation represents the most unfavorable derailed train speed. Solid elements are used to simulate the wheels of the train according to their actual size. The rims of the wheels are simulated as running inside the tracks to more accurately simulate actual conditions on the tracks. The train wheel size is given according to the size of the Chinese-manufactured train wheels. The contacts between wheels and tracks are shown in Figure 4. In MSC.Marc, the initial travel speed of the train can be defined through the Initial Conditions module. Han & Koo [17] showed that the coupler could be simulated with a link unit. Consequently, this work follows Han & Koo [17] method to simulate the coupler as a simple link, and the coupler will not break after the derailment.
|
|
|
(a) Front elevation of the contact between the wheelset and the track |
|
|
|
(b) Side elevation of the contact between the wheelset and the track |
|
Figure 4 The wheel-rail contact |
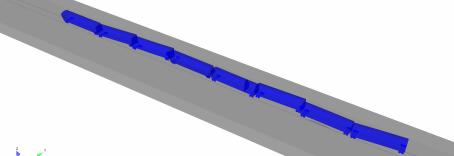
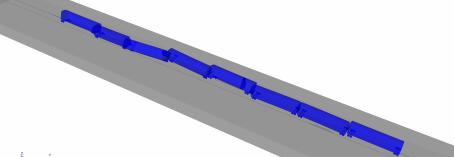
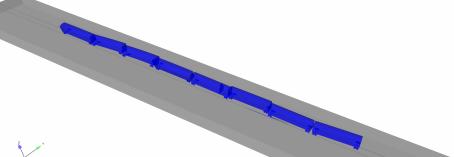
Solid elements are also used to model the car body to achieve an accurate simulation of the train-wheel-track-ground contact. The train models are simplified according to the simulation requirements. The train bogies are simplified using truss elements. The train models are shown in Figures 5 and 6. The lengths of carriages along the x-axis (Figure 5) are 15500 mm. The wheel diameter is 700mm.
|
|
|
Figure 5 Side elevation of the train model |
|
|
|
Figure 6 Plan view of the train model |
2.3 Boundary conditions
The bottom of the ground was fully fixed. The tracks were connected to the ground through sharing nodes in the finite element model. The entire model was subjected to gravity loads, which were assigned by defining the gravitational acceleration in a vertically downward direction.
2.4 Mesh generation and time step
The x-direction is the running (i.e., longitudinal) direction of the train; the y-direction is transverse to the running direction of the train; and the z-direction is the vertical direction. The sizes of mesh are as follows: the lengths of the elements along the y and z-axes (Figure 6) of the train are approximately 200 mm. The lengths of the elements along the x-axis (Figure 6) of the train are 200 mm to 500 mm. The size of the elements in the wheels is approximately 60 mm. The lengths of the elements along the x-, y-, and z- axis are 2000 mm, 73mm and 175 mm, respectively (Figure 3). This study adopts an implicit algorithm to solve the dynamic equations, and the time step is set to 0.250 ms.
2.5 The train derailment conditions
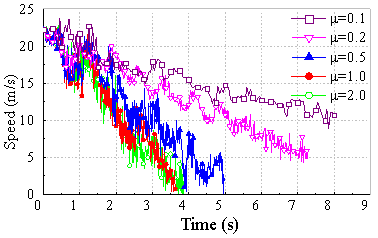
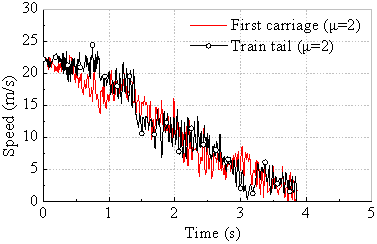
There are many potential causes of a train derailment because the actual behavior of the wheel-rail system is so complicated. Hence, this study introduces an artificial obstacle on the track, in front of the running train, to induce the train derailment. With this condition, the running attitude of the train after derailment can be simulated. The condition causing the train derailment is shown in Figure 7.