2.2 Constitutive relation of fibers

In THUFIBER program, the constitutive relation used for concrete fiber is shown as Figure 3. Users input 6 parameters to define the uni-axial stress-strain relation: the initial Young¡¯s Modulus of the concrete, the peak compressive strength f_c and the corresponding strain ¦Å_o, the ultimate compressive strength ¦Ò_u and the corresponding strain ¦Å_u, and the ultimate tensile strength f_t. The compressive constitutive model of concrete has a envelop curve containing two parts. The ascending part of the envelop curve is expressed by Equation 1, and the descending part is a straight line. The program can simulate confined and unconfined concrete behavior as well. The constitutive relation of rebar fiber is based on the L¨¦geron model and Bauschinger effect is also considered^[5], as shown in Figure 4.

             (1)

2.3 Test verification

THUFIBER program with the above mentioned constitutive models for the concrete and the rebar fiber agrees well in the dynamic simulation for the collapse experiment of RC planar frame ^[6], as shown in Figure 5. Figure 6 shows the relation between the axial force and displacement of the removed column. Obviously, the program has enough accuracy in collapse simulation. Besides, only 156 fiber beams with one integration point are used in dynamic time-history analysis which accomplishes within 1 minute on a general personal computer. That indicates great efficiency of fiber model.

3 Computational Models for Progressive Collapse

3.1 Geometric model

According to Chinese concrete code ^[7], an 8-stories RC frame is designed without special collapse design to check the collapse resistance of typical Chinese frames, as shown in Fig 7 and Fig 8. The ground storey is 4.2m high and all the other stories are 3.6m high. The sections of columns, longitudinal beams and transverse beams are 550¡Á550mm, 300mm¡Á500mm and 300mm¡Á550mm respectively. The columns have a fixed end on the ground.

3.2 Material parameters

The selected parameters for the materials are as follows. For concrete material, Young¡¯s Modulus E₀=30GPa, peak compressive strength f_c=30MPa, peak compressive strain ¦Å₀=0.002, ultimate compressive strength f_u=20MPa and ultimate compressive strain ¦Å_u=0.004. For the reinforcement, Young¡¯s Modulus E_s=200GPa, yield strength=335MPa and the broken tensile strain=0.1.

3.3 Slabs modeling

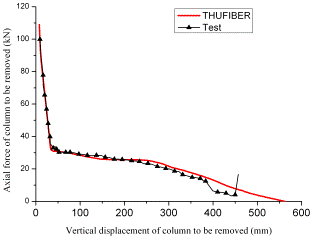
In order to study the influence of slabs on the progressive collapse behavior of RC frames, the numerical models of the frame with and without slabs are compared:

Model A: Frame model with columns and beams only;

Model B: Frame model including columns, beams and slabs. When progressive collapse happens, slabs are usually in large deformation stage, and therefore, the bending bearing capacity of slabs can be ignored comparing with their suspending capacity. So in this paper, the reinforcement in slabs is modeled with truss elements to consider the tensile effect of rebars, and the concrete in slabs is not taken into account. And moreover, when tensile strain of reinforcement reaches 10%, the reinforcement is considered to be broken, and then the procedure will delete it automatically. Thus, the contribution of slabs is conservatively considered by means of this method.

4 Analysis procedure

4.1 Load case

According to the specification in DoD2005 ^[2], the following factored load combination is applied to the entire structures for nonlinear dynamic analyses:

1.2G_k£«0.5Q_k£«0.2W_k                (2)

where, G_k =standard value of dead load; Q_k =standard value of live load; W_k =standard value of wind load.

4.2 Analysis procedure

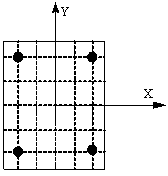
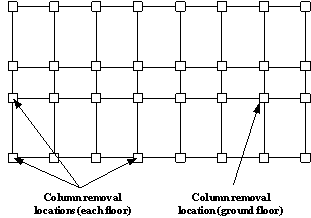
Alternate Path (AP) method based on the dynamic nonlinear analysis is used in this paper to analyze the progressive collapse resistance capacity because of its high accuracy. In AP method, one vertical load-bearing element is removed instantaneously from Model A and Model B in every load case to check the bridge over capacity of the residual structures. The columns are near the middle of the short side, near the middle of the long side and at the corner of the building at each floor are removed. Besides, an internal column at ground floor is also removed, as shown in Figure 9.      

According to the DoD2005 ^[2], the beams are considered to be failed (or collapsed) when their deflections reach 10% of their spans or the tensile strain of reinforcement in them exceeds 10%. If collapse area exceeds the value specified in DoD2005 ^[2], the structure will be considered that its progressive collapse resistance is insufficient and the collapsed part need be strengthened. Both the results of collapse resistance and strengthening reinforcement of Model A and Model B are compared to analysis the effect of slabs in collapse process.   

5 Results

5.1 Collapse resistance capacity

The results of simulations for collapse of Model A and Model B are listed in Table 1. It can be indicated that Chinese typical RC frames have insufficient collapse resistance. For the columns in different stories located at the same planar position, the columns in higher stories have more collapse possibility, as shown in Table 1, because the higher floor the removed column

Table 1 the results of simulations for collapse of Model A and Model B

locates, the less alternate loading paths the residual frame has. In addition, slabs enhance the resistance of progressive collapse (the examples marked by ¡°**¡±).

According to the simulation results as shown in Figure 10, it can be concluded that the tensile effect of slabs improves integrated load-bearing capacity which enhances the collapse resistance. Actually, the slabs still hold the broken beams though collapse happens, as shown in Figure 10(b).