1.      INTRODUCTION

With the rapid development of super-tall buildings (¡®super-tall¡¯ is defined as a building over 300 m in height by the Council on Tall Buildings and Urban Habitat [1]), the performance-based seismic design of super-tall buildings has become an important research issue in earthquake engineering. Numerous studies have been conducted in recent years using finite element (FE) models of super-tall buildings to simulate linear and nonlinear seismic response and to identify and understand potential collapse process. Lu et al. [2] established a refined FE model of the Shanghai World Financial Center (total height of approximately 492 m) using ANSYS. They analyzed the seismic response under different seismic intensities and compared the calculated response with the experimental data from a 1:50 scale shaking table tests. Fan et al. [3] also generated an FE model of Taipei 101 building (total height of approximately 508 m) with ANSYS and analyzed the seismic performance under various seismic load intensities. Lu et al. [4] established a refined FE model of the Shanghai Tower (total height of approximately 632 m) with MSC.Marc 2007 [5] and predicted the potential collapse process and collapse mode under extreme earthquakes. Jiang et al. [6] also built up an FE model of the Shanghai Tower with ABAQUS and analyzed the structural response and structural damage distribution under frequent earthquake, design level earthquake, and maximum considered earthquake (MCE) in an Intensity 7 Region specified in the Chinese Code for the Seismic Design of Buildings [7] . Similarly, Poon et al. [8] established FE model of the Shanghai Tower with Perform 3D and performed nonlinear dynamic time history analyses under seven sets of ground motions representing 2475-year return period earthquakes. 

It is currently a widely accepted practice to use FE models of super-tall buildings to predict their seismic response and to determine the potential collapse process. However, super-tall buildings have many different components resulting in an extremely large number of elements in the FE model. The computational workload required to create the refined FE model as well as the implementation of the subsequent time history analysis, parametric analysis or incremental dynamic analysis (IDA) are overwhelming. Therefore, it is necessary to establish a simplified model that can represent the key nonlinear characteristics of the super-tall building and can effectively reduce the modeling and computational effort.

Although limited research has been reported on establishing a simplified model of a super-tall building, many researchers have performed studies to establish simplified models of conventional tall buildings. For example, Connor & Pouangare [9] proposed a simplified model to design and analyze the elastic response of a framed-tube structure subjected to static lateral loads. Encina & de la Llera [10] introduced a wide-column model to simulate the modal and seismic history responses of the shear wall structures which have the free plan layout. Meftah et al. [11] presented a simplified method for the seismic analysis of an asymmetric building with uniform properties along its height, and the simplified formulas for the circular frequencies and internal forces subjected to earthquakes were obtained based on D¡¯Alembert¡¯s principle. Therefore, using an actual super-tall building as a case study (the Shanghai Tower), this paper establishes a simplified model of the super-tall building with nonlinear beam-column elements and nonlinear spring elements. The procedures are discussed in detail in the following sections, and the reliability of the simplified model is validated by comparing the results to the refined FE model through modal, static and time-history analyses.

On the other hand, energy-based design method has been widely used in structural seismic design [12-14]. One of the major goals of the seismic design is to effectively dissipate energy in targeted structural components or devices to ensure the safety of the entire building. In recent years, there has been a great deal of research performed on the plastic energy dissipation capacities of frame structures, shear wall structures and frame-shear wall structures. For example, Leger & Dussault [15] used equivalent viscous damping to represent the seismic energy dissipated by hysteretic behavior and other non-yielding mechanisms, evaluating the energy dissipation capacities of a series of multi-degree of freedom (MDOF) structures with different strength levels, strain hardening ratios and damping ratios. Lee & Bruneau [16] studied the energy dissipation capacity of compression members in concentrically braced frames using experimental data. The plastic energy dissipation capacities of steel beams suffering from ductile fracture under various loading histories were evaluated by Jiao et al. [17] . Similarly, Miao et al. [18] studied the energy dissipation mechanism in reinforced concrete frame-coupled shear wall structures with different span-depth ratios in coupling beams. For the booming super-tall buildings, the mega structural system is generally adopted and typically the dimensions of these components are unusually large. Hence, the mechanical characteristics of this structural system, the plastic energy dissipation of these very large structural components and the plastic energy distribution trends along the height of the buildings are still not clearly understood. Limited research has been reported on the plastic energy dissipation of the large structural components in super-tall buildings. Poon et al. [8] only provided a brief review of the energy time history curve of Shanghai Tower subjected to the Shanghai Synthesized record. Therefore, based on a simplified model of the Shanghai Tower, another major goal of this paper is to investigate and discuss the plastic energy dissipated by different components and total plastic energy distribution along the height of the building under three different seismic intensities (including the MCE in Intensity Regions 7, 7.5 and 8 in China) by employing time history analysis. This study intends to provide guidance and reference for further development of energy based design methods for super-tall buildings.

2.       SHANGHAI TOWER AND THE REFINED FINITE ELEMENT MODEL

Shanghai Tower, a multi-functional office building, is located next to two other super high-rise buildings in Lujiazui, Shanghai (Jin Mao Building and Shanghai World Financial Center). These three super high-rise buildings will be a new symbol of Shanghai. The three buildings are shown in Figure 1a. The total height of Shanghai Tower is about 632 m and the structural height is about 580 m with 124 stories above the ground level. A hybrid lateral-load-resisting system named ¡°mega-column/core-tube/outrigger¡± is adopted in Shanghai Tower and is shown in Figure 1b.

The mega column system contains eight mega columns extending from the bottom to the top of the building. The maximum cross section is about 5300 mm ¡Á 3700 mm and decreases to 2400 mm ¡Á 1900mm at the top. The core tube is made up of a 30 m ¡Á 30 m reinforced concrete (RC) tube, and the thickness of the concrete flange (outer) walls decrease gradually from 1.2 m at the bottom to 0.5 m at the top. Similarly, the thicknesses of the web (inner) walls decrease from 0.9 m at the bottom to 0.5 m at the top. In addition, the height of the coupling beams in the tube is about 1.0 m. Eight sets of two-story outrigger system are built at each mechanical/refuge story, which divide the entire structure into eight zones. The outrigger system consists the circle trusses and the outriggers with a total height of 9.9 m.

Because Shanghai Tower is located in a relatively high seismic region in China (with the maximum spectrum acceleration of 0.5g for the MCE level), both the wind and seismic loads play important roles in the structural design. The base shear force of the Shanghai Tower at the maximum design wind load is approximately 8.2¡Á10⁴ kN; while the seismic base shear force is approximately 8.8¡Á10⁴ kN (represents the 50-year return period earthquakes). The maximum story drift ratios of the Shanghai Tower for the maximum design wind and seismic loads are approximately 1/580 and 1/623, respectively. Both of them meet the limitation of the maximum story drift ratio, 1/500, at the design level. Since the damage of the Shanghai Tower will cause great economic loss and social impact, the seismic design objectives are relatively high. At the MCE level, the Shanghai Tower should only have moderately damage and the maximum story drift ratio shouldn¡¯t exceed 1/100. The local collapse as well as global collapse should be prevented. The failure of the joints connecting the key components should be avoided. The mega columns and core tube at the low zones should be immediate occupancy and those at the upper zones shouldn¡¯t exceed life safety. In addition, the shear failure of both the mega columns and core tube should be avoided. Yielding of the outriggers is permitted but the stress shouldn¡¯t exceed the ultimate strength [19] .

Using the general FE program MSC.Marc 2007, the three-dimensional FE model of Shanghai Tower is created by Lu et al.[4] . The final refined FE model of the building is shown in Figures 2a. It has approximately 120,000 elements and 441,000 degrees of freedom (DOF).

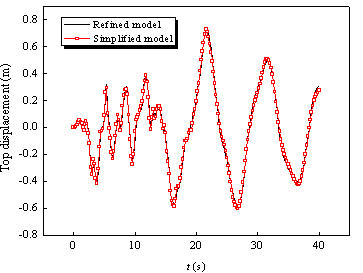
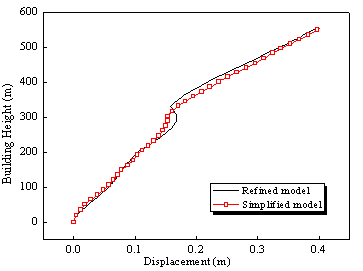
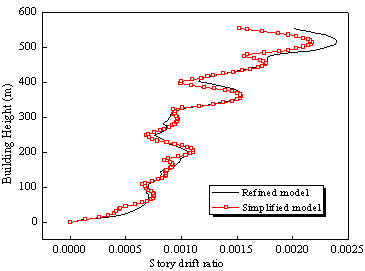
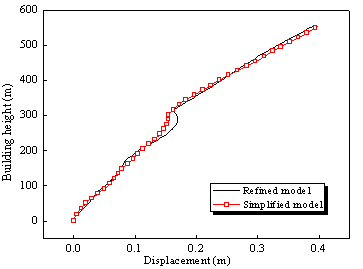
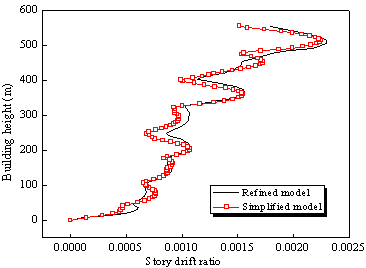
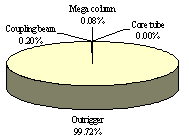
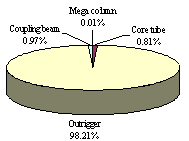
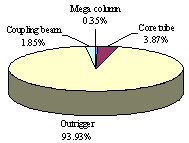
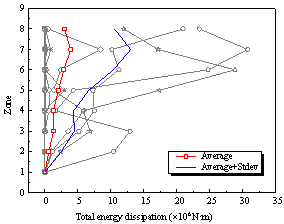
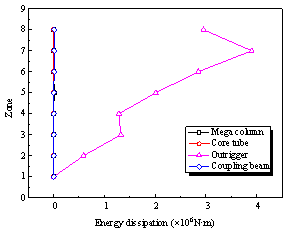
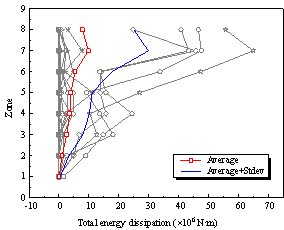
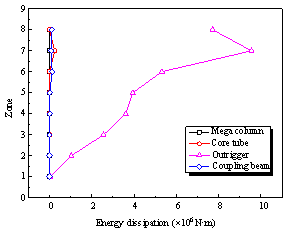
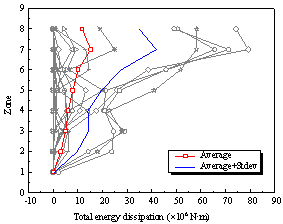
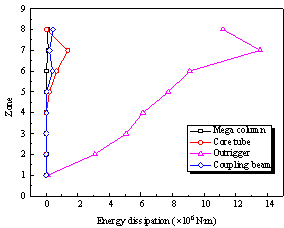
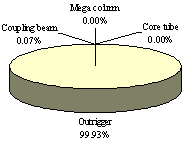
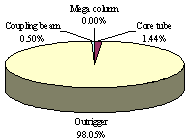
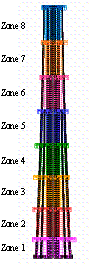3.      ESTABLISHMENT OF THE SIMPLIFIED MODEL

3.1   General provisions of the simplified model

To develop a simplified model of the Shanghai Tower, the following assumptions are made. The major objective was to characterize the plastic energy dissipation and dynamic properties of the structural components and system during seismic analysis.

(1) The three-dimensional (3D) refined FE model is used to develop the planar simplified model. The fundamental periods in the x and y directions of the 3D refined FE model are approximately 9.27 s and 9.19 s, respectively, indicating that the lateral stiffness in the two orthogonal directions is very similar. The first torsion period is approximately 4.09 s, and the torsion-translational period ratio is approximately 0.416 that is much smaller than 0.85 limit specified in the Technical Specification for Concrete Structures for Tall Buildings (JGJ 3-2010) [20] . It can be concluded that the significance of torsional effects in the Shanghai Tower is not predominant, and it is therefore feasible to simplify it into a planar model to primarily focus on the translational dynamic effects in seismic analysis.

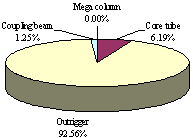
(2) The mega columns, core tube (including the shear walls and coupling beams) and the outrigger receive primary consideration in the simplified model and other components, such as secondary steel frames, are not included in the model. The selection of these components is based on the contribution of these components to the total lateral stiffness of the refined 3D FE model of the Shanghai Tower. The details of this procedure can be described as follows: To determine the stiffness contribution of various components in the refined FE model, the stiffness of each type of component (including the secondary frame, the mega column, the core tube and the outrigger) is reduced by 50%, while the total mass and all properties of other components remained unchanged. The first nine vibration periods of the reduced stiffness model and the original model are compared in Table 1. When the stiffness of the secondary steel frame is reduced the change in period is very small¡ªall are less than or equal to 0.35%. It can be concluded that the contribution of the secondary steel frame to the total structural lateral stiffness is negligible and can be ignored in the simplified model. When the stiffness of the mega columns is reduced by 50%, the change in translational periods is significantly large. The change in the fundamental period is approximately 15%, and it gradually decreases for higher vibration modes. However, the influence on the torsional periods is relatively small at approximately 2.58%. This suggests that the mega columns significantly contribute to the lateral stiffness of the entire building and this contribution is more obvious in the fundamental translational periods than in the higher mode periods. A 50% reduction in the stiffness of the core tube results in a large increase in all modal periods with more increase in the periods of higher vibration modes. The reduction in core tube stiffness also has a great impact on the torsional mode periods - an approximately 28% increase in torsional periods. These results indicate that the core tube stiffness has great contributions to both the structural lateral and torsional stiffness of the entire building. In addition, because the flexural deformation is predominant in the high order vibration modes and the core tube stiffness has significant impact on the flexural stiffness, the core tube stiffness contributes more to the stiffness of the higher order vibration modes. Finally, a reduction in the stiffness of the outrigger leads to a conclusion similar that of the mega columns. That is, the outrigger significantly contributes to the lateral stiffness of the building and has a smaller contribution to its torsional stiffness. Based on the above conclusions, the mega column, the core tube (including the shear wall and the coupling beam) and the outrigger are the primary components that need to be properly modeled in the simplified model of the Shanghai Tower.

(3) Nonlinear beam-column elements and nonlinear spring elements are adopted to model components in the simplified model. To make the simplified model easy to implement, the mega column, shear walls and the outrigger are simulated by nonlinear beam-column elements. Many research studies have been conducted to simulate columns and shear walls using nonlinear beam-column elements and springs under seismic loads. For example, Ibarra et al. [21] proposed a hysteretic model that incorporates strength and stiffness deterioration to simulate beams and columns. Subsequently, this model has been adopted to simulate the global collapse of frame structures subjected to earthquakes [22] , and it has been widely used. Rodrigues et al. [23] proposed a simplified hysteretic model to simulate the biaxial bending response of reinforced concrete columns. Setzler & Sezen [24] and Sezen & Chowdhury [25] simulated columns using flexural elements and nonlinear springs under lateral loads including the effect of strength and stiffness degradation that may be caused by shear or axial failure. Similarly, Orakcal & Wallace [26] used a beam-column element to simulate the flexural behavior of a reinforced concrete wall, and they also verified the rationality of this model with experimental data.

Table 1 Reduction in period of the original 3D model (%) when the component stiffness is reduced by 50% 

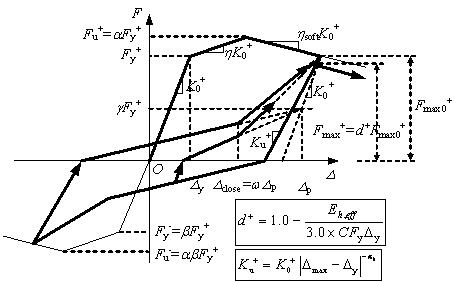
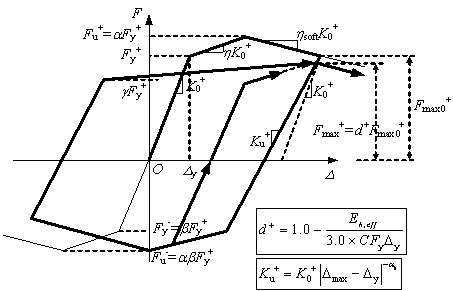
Because the planar simplified model is developed in this paper, the nonlinear beam-column element has three independent DOF (i.e., two translational DOF and one rotational DOF) with each DOF assigned to the corresponding cross-section-based hysteretic model. The hysteretic model proposed by Lu et al. [27] is adopted in this paper. The model is illustrated in Figure 3. The model considers not only the yielding, hardening and softening behavior but also the pinching and strength and stiffness deterioration effects under cyclic loading. Additionally, this model also demonstrates the phenomena of reversed yielding strength not equal to the positive yielding strength. The parameters in this model can be divided into two groups. The first group of parameters define the backbone curve, including K₀ (the initial stiffness), F_y (the generalized yielding strength, e.g., axial force, shear force, or bending moment), h (the hardening ratio), h_soft (the softening ratio), a (the ratio of peak strength and yield strength) and b (the ratio of reversed yield strength and positive yield strength). The second group of parameters controls the hysteretic behavior, including g (representing the pinching effect), w (representing the position of the ending point of slip), C (dimensionless accumulated hysteretic energy dissipation parameter, which reflects the capacity of resisting strength degradation caused by the cyclic loading.) and a_k (defining unloading stiffness). The values of these hysteretic parameters in the simplified model are obtained from numerical simulations and hysteretic component tests. Using a wide range of experimental data, Lu et al. [27] and Qu & Ye [28] showed that the hysteretic model can successfully simulate the nonlinear behavior of a variety of different components. Similarly, many simplified models have been proposed to simulate the cyclic response of coupling beams. For example, a beam element with two moment-hinges at the two ends and a shear-hinge in the middle was adopted to simulate the coupling beam in ATC 72 [29] . Therefore, in this paper, this model is used to simulate the coupling beam in the simplified model.

(4) The values of the hysteretic parameters for different components in the simplified model are determined using the refined FE model simulation results and the conventional component hysteretic tests. Because the dimensions of the components in the Shanghai Tower are very large, it is difficult to find similar component tests to calibrate the values of the hysteretic parameters. Therefore, the values of the hysteretic parameters in Figure 3 are determined according to the following two principles: (a) using the refined FE model with component dimensions, the force-displacement backbone curve under the cyclic lateral loads can be simulated, and then the backbone curve parameters (K₀, F_y, h, h_soft, a and b) can be obtained; (b) By taking into account the difficulties in simulating the hysteretic properties of the concrete components with the refined FE model, the values of the hysteretic parameters in Figure 3 (g, w, C and a_k) can be obtained from the conventional component hysteretic tests, if such experiments are reported in the literature. Due to the size effect of the conventional components in the tests, these parameters may not be very accurate, so a special parametric study is conducted later in this paper to show that the values of these hysteretic parameters do not have a significant impact on the overall simulation results.

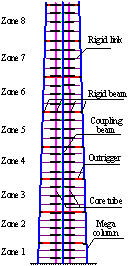
The final simplified model of the Shanghai Tower is established according to the above assumptions, and the model is shown in Figure 2b. Note that the 3D refined FE model has approximately 120,000 elements and 441,000 DOF, and the model size significantly decreases to approximately 2,500 elements and 7,200 DOF in the simplified model.

3.2   Simplified model for the mega column

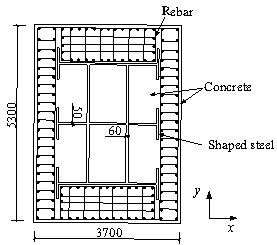
There are eight mega columns extending from the bottom to the top of the Shanghai Tower and the maximum cross section of the mega column is shown in Figure 4a. The mega column is constructed with concrete reinforced by rebar and shaped steel. The area of this mega column section is nearly 20 m² and has a steel ratio of 6.22% and a reinforcement ratio of 1.16%. The floor plan layout of these mega columns is shown in Figure 4b. Because these columns have similar strength and stiffness, the two mega columns on each side of the building are simplified and modeled as a single column as shown in Figure 4c. Results from the refined FE model showed that the main stress state of mega columns is bending and compression. Therefore, the nonlinear bending and compression behavior of the mega column is considered in the simplified model, while the shear behavior is simulated as elastic. The results from the refined FE model indicate that because the gravity load of the building is very large, the corresponding static axial forces on the mega columns are quite large. Therefore the axial force does not significantly change when subjected to the MCE. Therefore, the coupling effect between the axial force and the bending moment in mega columns can be disregarded. Thus, the bending moment-curvature relationship under compressive axial loads (due to gravity loading) is adopted to simulate the nonlinear flexural behavior of the mega column.

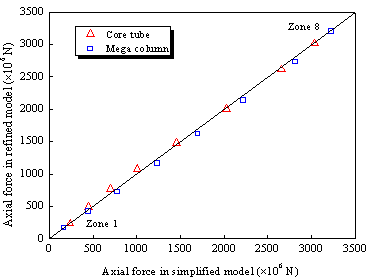
The refined FE models of mega columns from Zone 1 to Zone 8 (Figure 2a) are used to simulate the flexural behavior under given constant axial loads, and the moment-curvature backbone curves of these mega columns are obtained. One of the typical moment-curvature backbone curves of the mega column is shown in Figure 5. Because the steel-reinforced concrete mega columns in the Shanghai Tower have a maximum cross sectional area of approximately 20 m², it is difficult to determine the hysteretic performance from a full-scale experiments. Consequently, the hysteretic parameters of the mega columns in the simplified model are calibrated using the hysteretic test data from conventional steel-reinforced concrete columns, which have a similar steel ratio and axial load ratio to the actual mega columns in the Shanghai Tower. Chen et al. [30] conducted a hysteretic test of a steel-reinforced column with a 300 mm square cross section. The longitudinal steel ratio and the design axial load ratio were approximately 7.22% and 0.65, respectively, which are comparable to those of the actual mega columns in the Shanghai Tower. Therefore, the data from this hysteretic test of the steel-reinforced concrete column is used to calibrate the hysteretic parameters of the mega columns in the simplified model. The models shown in Figure 3 are used to simulate the hysteretic performance of this steel-reinforced column test. The results are compared in Figure 6, and the values of the hysteretic parameters of the mega column in the simplified model are determined.

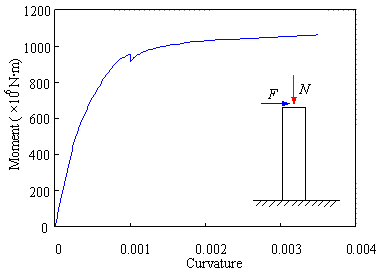
Figure 5 Typical bending moment-curvature backbone curve under constant compression for a single mega column

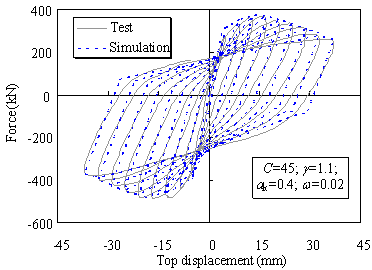
Figure 6 Comparison of the hysteretic test and simulation data for the steel reinforced concrete column

3.3   Simplified model for the coupling beam

In Shanghai Tower, steel plate reinforced composite coupling beams (i.e., a steel plate is embedded in the middle of the coupling beams) are used instead of the traditional reinforced concrete coupling beams to provide a higher stiffness and better ductility than the traditional RC coupling beams. The typical layout of coupling beams and walls in the core tube is shown in Figure 7. The distribution of the coupling beam is regular and mainly distributed symmetrically along the two orthogonal axes of the core tube. As the four coupling beams (referred to as A, B, C, and D in Figure 7) divide the core tube into two parts along the y axis, these four coupling beams are considered in the simplified model as one equivalent coupling beam in each story. Due to the constraint from the adjacent shear walls W₁, W₂ and W₃ (Figure 7), the two other coupling beams (i.e., E and F in Figure 7) are simulated as a part of the left or right half sub-tubes. Lu et al. [4] indicate that the failure of the coupling beam is primarily due to shear forces. Therefore, the coupling beam in the simplified model is simulated by a shear spring element as shown in Figure 8. The refined FE model of typical coupling beam in each story is developed, and the predicted backbone curve of the shear force-displacement relationship of a typical coupling beam is shown in Figure 9. This provides the backbone curve parameters of the hysteretic model used in the simplified model. The other hysteretic parameters are calibrated using steel plate reinforced composite coupling beam tests available in the existing literature. Lam et al. [31] conducted a series of hysteretic experiments on steel plate reinforced coupling beams with different configurations. The cross-section of one typical component was approximately 182 mm by 300 mm, and the thickness of the embedded steel plate was approximately 10 mm. The span-height ratio was approximately 2.5. The shear spring model shown in Figure 8 is adopted to simulate the hysteretic performance of the coupling beam, and the experimental and calculated responses are compared in Figure 10. The hysteretic parameters of the coupling beam can be determined from this comparison.

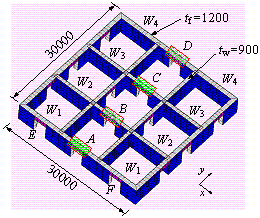
Figure 7 The distribution of the coupling beams in typical core tube (unit: mm)

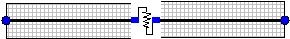
Figure 8 The simplified model of the coupling beam

Figure 9 The backbone curve of the coupling beam

Figure 10 Comparison of the hysteretic test and the simulated response of the steel plate reinforced coupling beam

3.4   Simplified model for the shear wall

The core tube is made up of a 30 m ¡Á 30 m RC tube; and the thickness of the concrete flange (outer) walls decreases gradually from 1.2 m at the bottom to 0.5 m at the top. Similarly, the thicknesses of the web (inner) walls decrease from 0.9 m at the bottom to 0.5 m at the top. The typical planar section of the tube is shown in Figure 7. As mention above, the layout of the coupling beams in the core tube is relatively regular and the four coupling beams along the y-axis (Figure 7) divide the core tube into two identical sub-tubes on the left and right side. These two sub-tubes are simulated by two cantilever beams in the simplified model and connected by coupling beams as described above. During the potential collapse process, the failure of the core tube is dominated by axial compression and bending [4] . In addition, similar to the mega column, due to the large gravity loads, the axial force variation in the core tube is insignificant under MCE loads. Thus, the moment-curvature relationship under constant gravity/axial loads is adopted to simulate the nonlinear flexural behavior of the core tube in the simplified model.

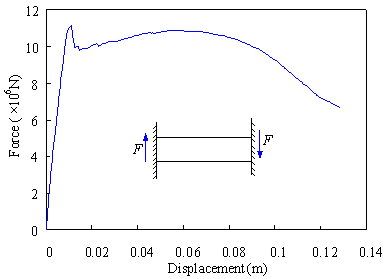
The refined FE model of the sub-tube in each of the eight zones is modeled one by one to simulate the flexural behavior under given axial loads and then the moment-curvature backbone curves of these tubes are obtained. A typical moment-curvature backbone curve is shown in Figure 11 and the corresponding backbone curve parameters are obtained. Hysteretic model parameters (Figure 3) are obtained using experimental data from reinforced concrete tube specimens tested under cyclic loads. Jia et al. [32] tested a series of core tubes with 1380 mm¡Á1380 mm overall dimensions, 70 mm thickness, and a design axial load ratio of approximately 0.5, which is comparable to that of the actual core tube in the Shanghai Tower. The beam-column element and hysteretic model in Figure 3 are adopted to simulate the hysteretic performance of the core tube specimen and to calibrate the hysteretic modeling parameters. The comparison of the experimental and simulated response and the obtained hysteretic parameters are shown in Figure 12.

Figure 11 Lateral load-displacement backbone curve for a typical core tube under axial compression

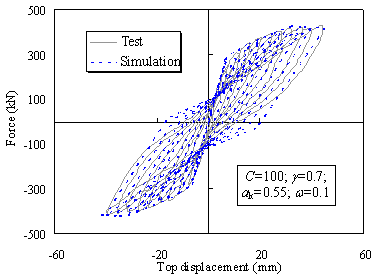
Figure 12 Comparison of the hysteretic test data and the simulated response of the core tube specimen

3.5   Simplified model for the outrigger

The outriggers in the Shanghai Tower, with a height of 9.9 m, are located at the mechanical and refuge story levels. The horizontal and diagonal members in the outrigger are made up of H-shaped steel. Main dimensions of the typical outrigger are shown in Figure 13. The outrigger is the major component connecting the inner core tube and the external mega columns, thus making the building work as a unit. The outrigger is designed mainly to resist the bending moment and the shear forces during an earthquake. The bending moment is primarily resisted by the upper and lower horizontal members (chords) while the shear force is generally withstood with diagonal members (Figure 13). Because the coupling effect between the bending moment and the shear force is not so obvious, a nonlinear flexural hinge and a shear hinge are adopted, respectively, to simulate the bending and shear behavior of the outrigger in the simplified model. First, a refined FE model of a typical outrigger is analyzed to obtain the backbone curves under bending moment and shear forces (Figure 14), and the backbone curve parameters are determined from this simulation. Because there are few reported studies of hysteretic tests of outriggers in the literature and the FE simulation of the steel components is relatively reliable, the hysteretic performance of the outrigger is also determined from the FE analysis. The cyclic shear responses obtained from the simplified model and the refined FE model are compared in Figure 15, and hysteretic modeling parameters are obtained. Each of the eight sets of outrigger systems located at the two-story mechanical/refuge stories consists of 20 outriggers with an angle q_i with respect to x-axis, as shown in Figure 16. The strength and stiffness of each outrigger is projected to the x-axis direction as a function of angle q_i.

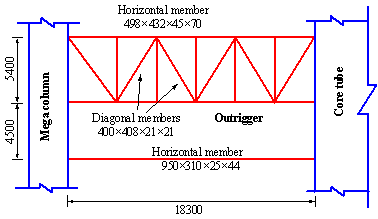
Figure 13 Details of a typical outrigger (unit: mm)

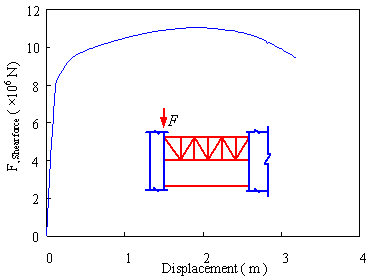
Figure 14 The backbone curve of the outrigger subjected to shear

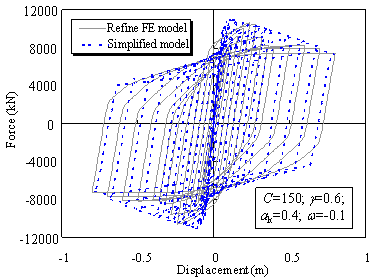
Figure 15 Cyclic shear response simulations of the simplified model and refined FE model of the outrigger

Figure 16 Spatial arrangements of the outriggers

3.6   Other modeling assumptions

The core tube and mega columns have a specified width in the refined FE model. In contrast, the simplified beam-column element cannot reflect this actual width in the simplified 2D model of the building. Therefore, rigid arms with a certain length are inserted at the junction between the mega columns and the core tube, as well as at the connections between the coupling beam and the shear wall in each story. The lengths of the rigid arms are determined from the actual width of the mega column or the core tube where the rigid arms are connected. Rigid links are inserted between the mega column and the core tube to represent the constraint of the floor slabs. Because the raft foundation with piles is adopted for the Shanghai Tower, which well constrains the displacement of the basement of the Shanghai Tower, both the refined FE model and the simplified model are fixed at the bottom. The soil-structure interaction effect and the energy dissipated by the adjacent soil are neglected in this paper. The final simplified model is shown in Figure 2b. Typical values of the hysteretic parameters for different components in Zone 1 are summarized in Table 2.

Table 2 Typical values of the hysteretic parameters of different components in Zone 1

4.      VALIDATION OF THE SIMPLIFIED MODEL

Based on the analytical results of the refined FE model, modal, static and dynamic time-history analyses are used to validate the simplified model. The first seven free translational vibration periods in the x-direction calculated from the refined and simplified models are shown in Table 3. The first seven free vibration periods of the two models are in good agreement, with a maximum relative error of approximately 3.76%. It can be concluded that the basic dynamic properties of the simplified model are reasonably close to those of the refined FE model.

Table 3 Comparison of the first seven periods (in seconds) from the simplified model and the refined FE model

Under the gravity loads, the total axial forces in mega columns and core tube at the bottom of each zone in the simplified and refined models are calculated and shown in Figure 17 within eight zones along the building height. A point on the diagonal line in the figure indicates that the axial force in that mega column or core tube in both models is equal. It can be seen that the axial forces in mega columns and core tubes in the simplified model agree well with the axial forces in the refined model.

Figure 17 Comparison of the axial force in mega columns and core tube of the simplified and refined models

The widely used ground motion recorded at El-Centro station in the United States in 1940 (referred to hereafter as ¡°El-Centro¡±) was chosen as a typical ground motion input in dynamic analyses. The peak ground acceleration (PGA) is scaled to 220 cm/s², which is the equivalent intensity of MCE for the Shanghai Tower. Comparisons of elastic displacement responses of the two models are shown in Figures 18 through 20. Figure 18 shows the two elastic roof displacement histories, which are almost identical. The elastic story displacement envelopes shown in Figure 19 and elastic story drift ratios shown in Figure 20 compare well, with the exception of consistent smaller drift ratios in the top zone in the simplified model. Overall, the 2D simplified model does a very good job of predicting the elastic seismic response compared to that predicted from the refined 3D FE model. Figures 17 through 20 show that the difference in response from the two models is well within the limits of acceptable engineering practices.

Figure 18 Comparison of the elastic roof displacement in the simplified model and the refined model

Figure 19 Comparison of the elastic story displacement envelopes for the simplified model and the refined model

Figure 20 Comparison of the elastic story drift ratio envelopes of the simplified model and the refined model

Figures 21 and 22 compare the nonlinear story displacement envelopes and story drift ratio envelopes, respectively, obtained from the simplified and refined FE models subjected to PGA= 220 cm/s² El-Centro ground motion. The predicted nonlinear displacement and drift responses agree reasonably well. The deviations in the responses near the middle height and top of the building are still within the acceptable range for practical engineering purposes. It can be concluded that, overall, the simplified model can accurately represent the basic dynamic characteristics of the Shanghai Tower and performs well in predicting the elastic and nonlinear seismic responses of the building.

Figure 21 Comparison of the nonlinear story displacement envelope between the simplified model and the refined model

Figure 22 Comparison of the nonlinear story drift ratio envelope between the simplified model and the refined model

5.      PLASTIC ENERGY DISSIPATION IN THE SHANGHAI TOWER

The cumulative hysteretic energy dissipated by structural members is a good indicator of structural performance under seismic loads. To control the structural damage during a strong earthquake, it is necessary to investigate the plastic energy dissipation interaction between various components and the hysteretic energy distribution throughout the building. This section focuses on the plastic energy dissipation ratios for different components and plastic energy distribution along the height of the Shanghai Tower under different seismic ground motions.

Due to the very large number of the elements used in the refined FE model of the Shanghai Tower, the corresponding computational workload is overwhelming. Therefore, the simplified model proposed above is used to evaluate the hysteretic energy dissipated by different components during nonlinear time-history analysis. The existing literature [4] [6] indicates that the well-designed Shanghai Tower has sufficient safety margins to meet the seismic demand for the MCE specified in the design code. Only 2 cm residual displacement is observed at the top of the building after the earthquakes with the PGA of 400 cm/s². To ensure that there are enough components of the Shanghai Tower entering the nonlinear stage, three different seismic intensities are used: PGA=220 cm/s² (i.e., the MCE in the Intensity 7 Region, which is the specified design intensity for the Shanghai Tower); PGA=310 cm/s² (the MCE in an Intensity 7.5 Region); and PGA=400 cm/s² (the MCE in Intensity 8 Region). Due to the significant randomness in the input ground motion records, the seismic response obtained from a single specified ground motion record may result in a large deviation. Therefore, 22 far-field ground motions suggested by the FEMA P695 [33] are adopted as the basic ground motion set. The classical Rayleigh damping model with the damping ratio of 5%, which specified in the Specification for the Design of Steel-Concrete Mixed Structure in Tall Buildings [34] , is adopted in the analysis. The plastic energy dissipation contribution of different components and the plastic energy dissipation distribution along the building height are discussed based on the mean value of the response parameters obtained from the 22 ground motions.

The above-mentioned 3 PGA intensities and the 22 ground motion records generate 66 input motions for the nonlinear time history analysis. The average results indicate that the plastic energy dissipated by the structural components in the Shanghai Tower are 21.37%, 26.25% and 29.09% of the total seismic input energy when the earthquake PGA is equal to 220 cm/s², 310 cm/s² and 400 cm/s², respectively. The ratios of plastic energy dissipated by each major component of the main lateral load resisting system (i.e., the mega columns, core tube, outriggers and coupling beams) are shown in Figure 23 for the three different seismic intensities considered. When PGA=220 cm/s², the mega columns and the core tube primarily remain elastic, with only few coupling beams and outriggers entering into nonlinear stage. Almost the entire plastic energy, or 99.72% of it, was dissipated by the outriggers. When the intensity increases to PGA=310 cm/s², parts of the core tube also exhibit very limited nonlinear response. The plastic energy dissipation contribution of the coupling beams increases to 0.97%, and the plastic energy dissipation contribution of outriggers decreases slightly to 98.21%. However, the outriggers are still the primary plastic energy dissipation component. When the earthquake intensity increases to PGA=400 cm/s², all components of the lateral loading resisting system enter into nonlinear range. The plastic energy dissipation contributions of the coupling beams, core tube and mega columns greatly increase, while the plastic energy dissipation in the outriggers decreases slightly. The outriggers are still the primary plastic energy dissipation component in the Shanghai Tower, with a proportion of 93.93%. The average shear displacement ductility of the steel outrigger is approximately 10. Note that this shear displacement is the relative vertical displacement between the two ends of the outrigger. Apart from bearing the lateral seismic loads, the mega columns and core tube are also the main components for resisting the vertical gravity load. These are the key components for resisting structural collapse and should not be adopted as the major plastic energy dissipation components. As a result, the coupling beams and outriggers are the potential plastic energy dissipation components in the Shanghai Tower. Figure 23 clearly shows that even when subjected to three different seismic intensities, the contribution of outriggers to the total plastic energy dissipation is much greater than that of the coupling beams. Thus, the outrigger is the major plastic energy dissipation component in the Shanghai Tower.  

The total plastic hysteretic energy dissipation distribution and the average plastic energy dissipation contribution of each component along the building height are shown in Figures 24 to 25. In Figure 24a, the gray lines represent the distribution of the total hysteretic energy dissipation along the building height for each specified ground motion. The red-scattered line represents the average total plastic energy dissipation for the 22 ground motions, and the blue-solid line is the average plus one time the standard deviation. The structural hysteretic energy dissipation has a large standard deviation. Since the higher-order modes play an important role in the super-tall building¡¯s seismic response, the damage degree in the upper zones of the building is more serious than in the lower zones. Consequently, the hysteretic energy dissipation is mainly concentrated in the upper zones of the building and gradually decreases with the decreasing of the building height. Similarly, Figures 24b, 25b and 26b also indicate that the hysteretic energy dissipation in each structural component increases gradually with increasing height and is mainly distributed within the top four zones. Figures 23 through 26 also illustrate that the hysteretic energy dissipation is mainly concentrated in the outriggers and increases with increasing seismic intensity. Although the plastic energy dissipation contribution of the outriggers decreases slightly, it is still the major plastic energy dissipation component in the Shanghai Tower (Figure 23). In addition, the analysis indicates that the primary hysteretic energy dissipation in the outriggers is due to the yielding of the diagonal components.

Because the hysteretic modeling parameters of the components in the above analysis are determined from similar conventional structural component experiments without considering the size effect, the assigned values may not be very accurate. To discuss the influence of hysteretic parameters on the system energy dissipation, the hysteretic parameters (C, g,   a_k, and w) suggested in Section 2 are modified and the values of these modified parameters are shown in Table 4. Using the modified hysteretic parameters in the simplified model, the above-mentioned three analysis cases are repeated. The new average plastic energy dissipation contribution of each component is provided in Figure 27. By comparing Figures 23 and 27, it can be concluded that no major change was observed in contribution of components to the plastic energy dissipation when the hysteretic parameters are changed. The outrigger, which is the major plastic energy dissipation component, still accounts for the major portion of the total hysteretic energy dissipation in the Shanghai Tower.

Tables 4 Modified hysteretic modeling parameters

6.      CONCLUSIONS

A simplified model is developed for seismic analysis of super-tall buildings using the Shanghai Tower as a case study building. The two-dimensional simplified model includes nonlinear beam-column elements and shear spring elements. The following conclusions are reached as a result of this investigation:

(1) The proposed simplified model of the Shanghai Tower represents the basic dynamic characteristics of the building and predicts the elastic and nonlinear seismic response well. This study provides guidance for the development of a simplified model that can be used for dynamic response simulation other super-tall buildings. Seismic analysis of a super-tall building can be performed efficiently and much faster using the proposed two-dimensional simplified frame model with beam and spring elements than a three-dimensional finite element model.

(2) The outrigger is the primary plastic energy dissipation component in the Shanghai Tower. With increasing seismic load intensity, the plastic energy dissipation contribution of coupling beams increases slightly and the core tube begins to participate in plastic energy dissipation. Although the plastic energy dissipation contribution of the outrigger decreases slightly under larger seismic loads, it is still the major plastic energy dissipation component in the building.

(3) Because the higher-order vibration modes play an important role in seismic behavior of a super-tall building, more plastic energy is dissipated near the top of the building, i.e., in the upper four zones of the Shanghai Tower. Correspondingly, the plastic energy dissipation in outriggers located in the upper four zones is much greater than outriggers in the lower zones.

(4) The hysteretic law and the associated modeling properties have negligible influence on the contribution of different components to the total plastic energy dissipation.

ACKNOWLEDGMENT

The authors are grateful for the financial support received from the National Nature Science Foundation of China (No. 51222804, 91315301, 51261120377), the Beijing Natural Science Foundation (No. 8142024) and the Fok Ying Dong Education Foundation (No. 131071). In addition, the third author acknowledges the support from the Tsinghua University Initiative Scientific Research Program (No. 2011THZ03) for his visit to Tsinghua University. The second and fourth authors¡¯ visit to the Ohio State University was also funded through the National Nature Science Foundation of China (No. 51222804). This support is greatly appreciated.

REFERENCES£º

[1]       CTBUH Height Criteria. Council on tall buildings and urban habitat. http://www.ctbuh.org/HighRiseInfo/TallestDatabase/Criteria/tabid/446/language/en-US/Default.aspx. [Retrieved 7 December 2011].

[2]       Lu XL, Zhu JJ, Zou Y. Study on performance-based seismic design of Shanghai World Financial Center Tower.          J. Earthq. Tsunami. 3/04 (2009) 273-284.

[3]       Fan H, Li QS, Tuan Alex Y, Xu LH. Seismic analysis of the world's tallest building, J. Constr. Steel Res. 65/5 (2009) 1206-1215.

[4]       Lu X, Lu XZ, Zhang WK, Ye LP. Collapse simulation of a super high-rise building subjected to extremely strong earthquakes, Sci. China Tech. Sci. 54/10 (2011) 2549-2560.

[5]       MSC. Software Corp. MSC. MARC. User¡¯s manual. MSC Software Corporation, Santa Ana, CA. 2007.

[6]       Jiang HJ, He LS, Lu XL, Ding JM, Zhao X. Analysis of seismic performance and shaking table tests of the Shanghai Tower, J. Build. Struct. 32/11 (2011) 55-63. (in Chinese)

[7]       GB50011-2010, Code for Seismic Design of Buildings, Beijing: Ministry of Housing and Urban-Rural Development of the People¡¯s Republic of China, 2010.

[8]       Poon DCK, Hsiao LE, Zhu Y, Leonard J, Zuo S, Fu G, Ihtiyar O. Non-linear time history analysis for the performance based design of Shanghai Tower, In: ASCE Conference Proceedings, Las Vegas, Nevada, USA 401/47, (2011). doi:http://dx.doi.org/10.1061/41171(401)47.

[9]       Connor JJ, Pouangare CC. Simple-model for design of framed-tube structures, J. Struct. Eng.-ASCE. 117/12 (1991) 3623-3644.

[10]    Encina J, de la Llera JC. A simplified model for the analysis of free plan buildings using a wide-column model. Eng. Struct. 56 (2013) 738-748.

[11]    Meftah SA, Tounsi A, El Abbas AB. A simplified approach for seismic calculation of a tall building braced by shear walls and thin-walled open section structures, Eng. Struct. 29/10 (2007) 2576-2585.

[12]    Leelataviwat S, Goel SC, Stojadinovic B. Energy-based seismic design of structures using yield mechanism and target drift. J Struct. Eng.-ASCE. 128/8 (2002) 1046¨C1054.

[13]    Choi H, Kim J. Energy-based seismic design of buckling-restrained braced frames using hysteretic energy spectrum. Eng. Struct. 28/2 (2006) 304-311.

[14]    Habibi A, Chan RWK, Albermani F. Energy-based design method for seismic retrofitting with passive energy dissipation systems. Eng. Struct. 46 (2013) 77-86.

[15]    Leger P, Dussault S. Seismic-energy dissipation in MDOF structures, J. Struct. Eng.-ASCE. 118/5 (1992) 1251-1269.

[16]    Lee K, Bruneau M. Energy dissipation of compression members in concentrically braced frames: Review of experimental data, J. Struct. Eng.-ASCE. 131/4 (2005) 552-559.

[17]    Jiao Y, Yamada S, Kishiki S, Shimada Y. Evaluation of plastic energy dissipation capacity of steel beams suffering ductile fracture under various loading histories, Earthq. Eng. Struct. D. 40/14 (2011) 1553-1570.

[18]    Miao ZW, Qiu ZY, Ming Y. Study on energy dissipation mechanism and collapse-resistant performance of RC frame-shear-wall structure under strong earthquake. Appl. Mech. Mater. 204 (2012) 2550-2554.

[19]    Jiang HJ, Lu XL, Zhao X. Performance-based seismic design principles and objectives of Shanghai Tower. Proceedings of IABSE-IASS 2011 Symposium, Paper No. P0121, September 20-23, 2011, London, UK.

[20]    JGJ 3-2010, Technical specification for concrete structures of tall building, Beijing: Ministry of Construction of the People¡¯s Republic of China, Beijing, China, 2010. (in Chinese)

[21]    Ibarra LF, Medina RA, Krawinkler H. Hysteretic models that incorporate strength and stiffness deterioration, Earthq. Eng. Struct. D. 34/12 (2005) 1489-1511.

[22]    Ibarra LF, Krawinkler H. Global Collapse of Frame Structures under Seismic Excitations. PEER Report 2005/06, Berkeley, CA, USA.

[23]    Rodrigues H, Romão X, Andrade-Campos A, Varum H, Arede A and Costa, AG. Simplified hysteretic model for the representation of the biaxial bending response of RC columns. Eng. Struct., 44 (2012) 146-158.

[24]    Setzler EJ, Sezen H. Model for the lateral behavior of reinforced concrete columns including shear deformations, Earthq. Spectra. 24/2 (2008) 493-511.

[25]    Sezen H, Chowdhury T. Hysteretic model for reinforced concrete columns including the effect of shear and axial load failure, J. Struct. Eng.-ASCE. 135/2 (2009) 139-146.

[26]    Orakcal K, Wallace JW. Flexural modeling of reinforced concrete walls: Experimental verification, ACI Struct. J. 103/2 (2006) 196-206.

[27]    Lu XZ, Ye LP, Miao ZW. Elasto-plastic analysis of buildings against earthquake, Beijing: China Architecture & Building Press, 2009. (in Chinese)

[28]    Qu Z, Ye LP. Strength deterioration model based on effective hysteretic energy dissipation for RC members under cyclic loading, In: Proc. 7th Intl. Conf. on Urban Earthquake Eng.(7CUEE) & 5th Intl. Conf. on Earthquake Eng.(5ICEE), Tokyo, Japan, (2010) pp. 851-856.

[29]    PEER/ATC-72-1: Modeling and Acceptance Criteria for Seismic Design and Analysis of Tall Buildings, Applied Technology Council, Council, Redwood City, CA. 2010.

[30]    Chen XG, Mu ZG, Zhang JB, Wang CK, Chen CH, Sun HZ. Experimental study on the seismic behavior of steel reinforced concrete columns, J. Univ. Sci. Tech. Beijing 31/12 (2009) 1516-1524. (in Chinese)

[31]    Lam WY, Su RKL, Pam HJ. Experimental study on embedded steel plate composite coupling beams, J. Struct. Eng.-ASCE 131/8 (2005) 1294-1302.

[32]    Jia P, Du XL, Zhao J. Seismic behavior of reinforced concrete core wall under different axial load ratio, J. Beijing Univ. Tech. 35/1 (2009) 63-69. (in Chinese)

[33]    FEMA P695: Quantification of building seismic performance factors, Applied Technology Council, Redwood City, CA. 2009.

[34]    CECS 230-2008. Specification for Design of Steel-concrete Mixed Structure of Tall Buildings. Beijing: China Institute of Building Standard Design & Research, 2008 (in Chinese)