2. Experimental program
2.1 Design of the specimens
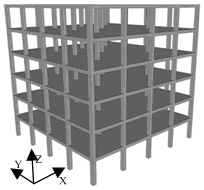
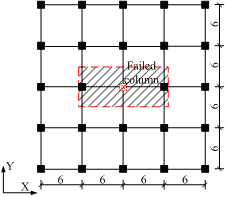
The prototype structure is a six-story RC frame (Figure 1) designed in accordance with the Chinese building codes [30,31]. The first story is 4.2 m in height, and the remaining stories are 3.6 m in height. The span length in both directions is 6 m. The design dead and live loads are 5.0 kN/m2 and 2.0 kN/m2, respectively. The beams and columns in the structure are designed to be ductile-bending-controlled while the shear and torsional failure can be prevented [30,31]. According to the code requirement [30,31], the beams and columns are expected to be damaged in bending in which the tension reinforcement yields while concrete in compression crushes, resulting in a large ductile rotational deformation. The beam-slab substructure being tested is highlighted with the shaded area enveloped by the red rectangle, as shown in Figure 1b. Due to the restraint of the laboratory space, the substructure was scaled down to 1/3. Published research confirmed that the critical scaling factor for RC specimens not damaging in shear is 1/4 which can well represent the resistance mechanisms and load-displacement relations of large scaled specimens [32]. Hence, 1/4 [16,24], 1/3 [17,21-23] and 1/2 [19,20] scales were adopted in many progressive collapse tests on RC substructures, in which the size effect on the collapse mechanism and resistance can be neglected [20,32]. The sectional dimensions of the prototype structure and the control specimen are given in Table 1. The thicknesses of the concrete cover of the beams and slabs of the test specimens were 6 mm and 5 mm, respectively.
Table 1 Sectional sizes of the prototype structure and the control specimen
|
Type |
Column |
Beam |
Boundary beam |
|
Prototype structure |
600 mm × 600 mm |
250 mm × 500 mm |
— |
|
Control specimen |
200 mm × 200 mm |
85 mm × 170 mm |
500 mm × 370 mm |
|
|
|
|
(a) Perspective view |
(b) Plan view |
|
Figure 1 The prototype structure (units: m) |
|
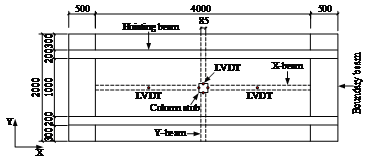
The tested area within the real structure was restrained along the perimeter edges by the surrounding slabs and beams. In the tests, the actual boundary condition was simplified as having two fixed sides in the x-direction and two free sides in the y-direction. This represented a one-way beam-slab substructure. Although, in reality, the slabs are restrained in both directions resulting in two-way CMA and TMA, one-way CMA and TMA being the basis of the two-way actions have not previously been studied systematically. Hence the one-way actions were investigated in this work to provide the fundamental understanding for further studies on the two-way actions. To reliably achieve the required one-way boundary condition, two strong boundary beams were designed to be part of the specimens (Figure 2). These boundary beams had a much larger sectional size than the structural beams. Therefore they had sufficient stiffness to restrain the translation and rotation of the specimen and provided adequate space in which the longitudinal reinforcement of the specimen could be well anchored. In addition, to facilitate the transportation and lifting of the specimens, two hoisting beams were cast monolithically with each specimen. The hoisting beams were able to support the weight of the specimens to avoid damage to the specimens during the transportation and lifting process. At the position of the removed column, a concrete stub (with a sectional dimension shown in Table 1) was designed to have a 100 mm extrusion from the beam soffit (Figure 2b).
|
|
|
(a) Plan view |
|
|
|
(b) Elevation view |
|
Figure 2 Dimensions of the control specimen (units: mm) |
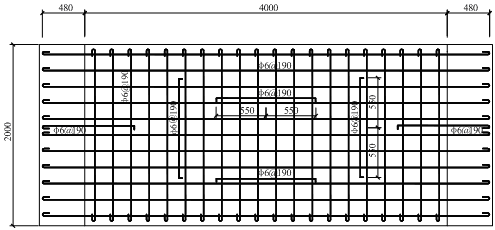
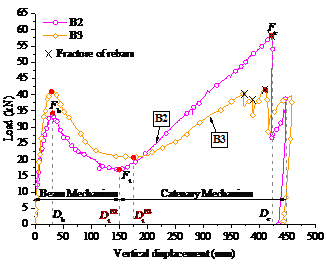
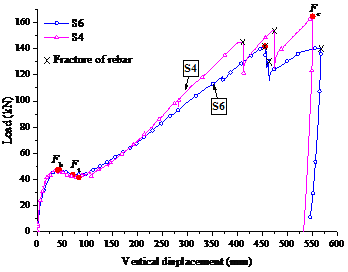
A total of seven specimens were designed to study the effects of various structural parameters on the collapse resistance. These included five beam-slab substructure specimens (referred to as the “S-series” and designated as S2 to S6) and two continuous-beam specimens without slabs (referred to as the “B-series” and designated as B2 and B3). The sectional sizes and the reinforcement details are given in Table 2 and Figure 3. The structural parameters considered were the slab thickness ts, the slab width ws, the beam height hb, and the seismic reinforcement. Specimens S6 and B2 were the control specimens for the S- and B-series, respectively. Their seismic design intensity was 6 degree, i.e., the peak ground acceleration (PGA) was 0.05 g for the design earthquake (with a 10% probability of exceedance in 50 years), where g was the acceleration of gravity. The other specimens differed from S6 (or B2) by altering only one parameter. For instance, the slab width of S2 was changed to the effective flange width as specified in the design code [30] (i.e., six times the slab thickness on each side of the beam), instead of 1 m on each side of the beam axis. S3 (B3) had a larger beam height of 200 mm, compared to the height of S6 (B2), which was 170 mm. S4 had a higher seismic design intensity of 8 degree (PGA = 0.20 g for the design earthquake), i.e. a higher level of earthquake demand, which resulted in a larger amount of seismic reinforcement for the beam (Table 2). S5 had a larger slab thickness of 75 mm. In consequence, to satisfy the requirement for the minimum reinforcement ratio of the slab specified in the design code [30], the amount of slab reinforcement in S5 was also increased (Table 2).
Table 2 Cross-sectional dimensions and reinforcement details (units: mm)
|
Specimen |
Beam height hb |
Beam width wb |
Slab thickness ts |
Slab width ws |
Beam top |
Beam bottom |
Slab bottom |
Slab top |
|
|
Beam end |
Mid-span |
Longitudinal / Transverse |
Longitudinal / Transverse |
||||||
|
B2 |
170 |
85 |
— |
— |
2f8+1f6 |
2f8 |
2f8 |
— |
— |
|
B3 |
200 |
85 |
— |
— |
2f8+1f6 |
2f8 |
2f8 |
— |
— |
|
S2 |
170 |
85 |
50 |
685 |
2f8+1f6 |
2f8 |
2f8 |
f 6@190 |
f 6@190 |
|
S3 |
200 |
85 |
50 |
2000 |
2f8+1f6 |
2f8 |
2f8 |
f 6@190 |
f 6@190 |
|
S4 |
170 |
85 |
50 |
2000 |
3f10 |
2f10 |
2f10 |
f 6@190 |
f 6@190 |
|
S5 |
170 |
85 |
75 |
2000 |
2f8+1f6 |
2f8 |
2f8 |
f 6@160 |
f 6@160 |
|
S6 |
170 |
85 |
50 |
2000 |
2f8+1f6 |
2f8 |
2f8 |
f 6@190 |
f 6@190 |
|
|
|
|
(a) Reinforcement in the slab |
|
|
|
|
|
(b) Reinforcement in the X-beam |
|
|
|
|
|
(c) Reinforcement in the Y-beam |
(d) Sectional view of the beam |
|
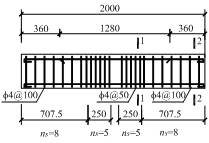
Figure 3 Details of the reinforcement in S6 (units: mm) |
|
Note: ns is the number of steel hoops in the corresponding area.
The reinforcement ratios for the longitudinal tension reinforcements of B2, S2, S6 and S5, B3 and S3, and S4 were 1.0%, 0.8% and 1.7% respectively which were between the minimum and maximum ratios (i.e. 0.2% and 2.5%, respectively) regulated by the Chinese codes [30,31]. According to these codes [30,31], the amount of transverse reinforcement in beams is determined by the calculated seismic shear demand and detailing requirements of stirrup reinforcement. For the prototype structures with two different seismic safety levels (i.e. the seismic design intensities), the seismic shear demands are already satisfied when the stirrups meet the detailing requirements for the corresponding seismic safety levels. In other words, the amount of stirrups regulated by the detailing requirements specified in the codes [30,31] is related to the geometrical dimensions of the beams. This resulted in a similar amount of transverse reinforcement in the beams of the specimens. For example, the spaces of the stirrups in the plastic hinge region were supposed to be 48mm for B3 and S3 and 43mm for the other specimens respectively, according to the requirements in the Chinese codes [30,31]. The stirrup spacing in the other regions should be 150mm for S4 and 90mm for the other specimens, respectively [30]. Given that the diameter of immersion vibrators is approximately 50mm, to facilitate vibration of concrete, 50mm and 100mm were chosen in this work as the stirrup spacing in the plastic hinge regions and the other regions, respectively. As no shear failure was observed in the test, such a spacing was considered satisfactory. The reinforcement ratios for the stirrups inside and outside the plastic hinge regions were 0.6% and 0.3% respectively which were larger than the minimum ratio 0.24ft/fvy (i.e. 0.17%) regulated by the Chinses code [30], where ft was the tensile strength of the concrete and fvy was the yield strength of the stirrups.
2.2 Material properties
The specimens were made with C30-grade concrete, with an average compressive strength of 44 MPa, which was determined using the standard cubes with a size of 150 mm × 150 mm × 150 mm. The average yield strengths of the rebars with diameters of 4 mm, 6 mm, 8 mm, and 10 mm were 618 MPa, 387 MPa, 390 MPa, and 370 MPa, respectively, and the average ultimate strengths were 715 MPa, 475 MPa, 468 MPa, and 560 MPa, respectively.
2.3 Test setup and instrumentation
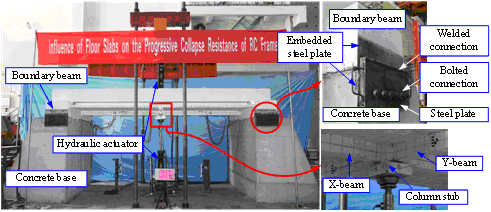
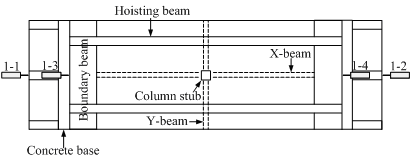
To achieve the fixed boundary condition at the two supports in the x-direction and provide enough space for large deformation of the specimens, two large concrete bases fixed on the strong ground floor were placed under the boundary beams (Figure 4). For each pair of the boundary beam and the connecting concrete base, two sets of two steel plates (the steel plate in the boundary beam was 500 mm × 160mm × 20mm whilst that in the concrete base was 500 mm × 230 mm × 35 mm) were embedded in the vicinity of their front and back surfaces (see Figure 4). When a specimen was placed on the two bases, an external steel plate with a dimension of 500 mm × 260 mm ×20 mm was welded and bolted, respectively, to the embedded plates in the boundary beam and the concrete base (Figure 4a). By doing so, fixed connections between the boundary beams and the concrete bases could be achieved. The linear variable differential transducers (LVDTs) that were installed at the boundary beams and at the concrete bases, shown as 1-1 through 1-4 in Figure 5, further confirmed the fixity of the boundary condition during the test.
|
|
|
|
(a) Front view |
|
|
|
|
|
(b) Front perspective view |
(c) Back perspective view |
Figure 4 Test setup
Concentrated vertical loads were applied to
each specimen at the position of the removed column using two hydraulic actuators,
one above and the other below the specimen (Figure 4a). At the beginning of
each test, a pair of small balanced forces (< 10 kN) was simultaneously
applied by the two actuators. Afterwards, while the lower actuator maintained
the small constant force, the upper actuator applied a gradually increased
force. The displacement-controlled loading method was adopted in the test
with a loading rate of 2 mm/minute and 4 mm/minute under the beam and caternary
mechanisms, respectively. Thereby, a continuously increased deformation was
applied to the specimens by which the structural resistance of the specimens
at different deformation stages were obtained after the removal of a middle
column. In addition, a stable loading process could also be achieved by this
loading approach when the stiffness and strength of the specimens significantly
deteriorated under large deformation.
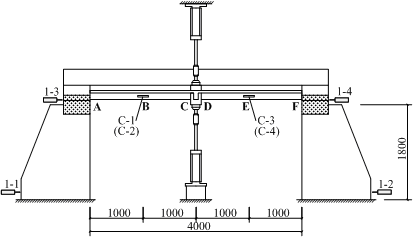
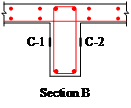
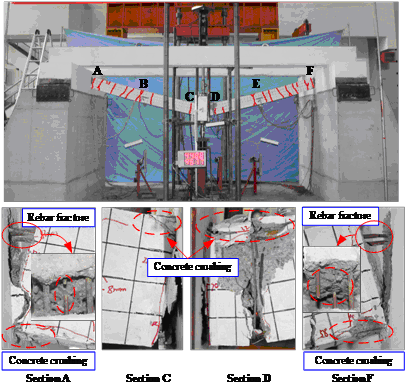
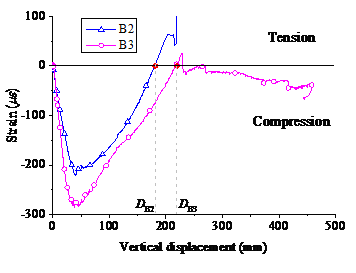
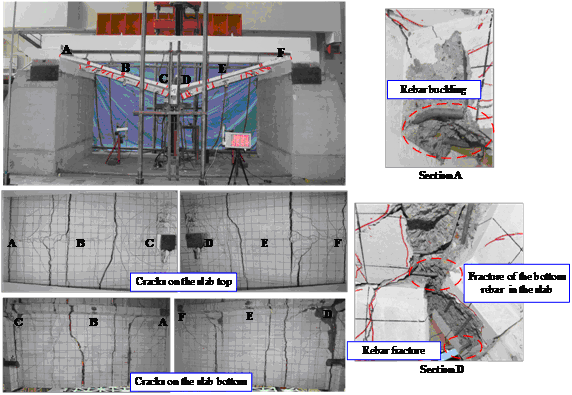
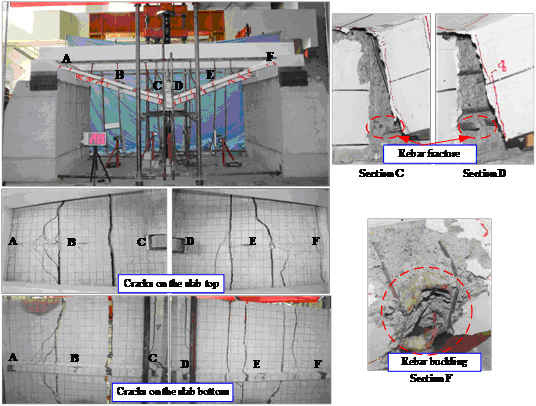
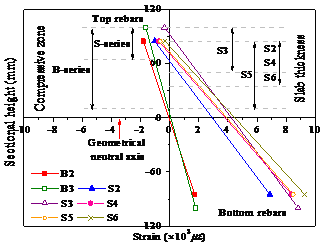
Six typical sections along the beam are defined as Sections A through F, as shown in Figure 5. To measure the displacements at the critical locations, LVDTs were installed at the four corners of the concrete stub and at the mid-span of each beam (i.e., Sections B and E) (Figure 2a). To monitor the strains within the specimens, strain gauges were placed to the steel bars in the beams and slabs at Sections A through F. In addition, strain gauges in concrete were installed at the mid-heights of Sections B and E, shown as C-1 through C-4 in Figure 5.
|
|
(c) Locations of the concrete strain gauges |
|
(a) Elevation view |
|
|
|
|
|
(b) Plan view |
Note: Labels A through F mean the critical sections of the beam.
Figure 5 Details of test setup and arrangement of instrumentation (units: mm)